www.industry-vietnam.com
25
'09
Written on Modified on
Exploring Chaos and Dynamical Systems with MapleSim
Dr. Stephen Lynch is a world class leader in the use of mathematical software in teaching and learning, and a renowned expert on dynamical systems and their applications. He teaches a range of courses at Manchester Metropolitan University in the UK, and has been nominated for a second time by MMU for a National Teaching Fellowship in recognition of his excellence in teaching at the higher education level.
Dr. Lynch has chosen to feature MapleSim in the second edition of his best-selling book, Dynamical Systems and Applications using Maple. Due to be published in the summer of 2009, the book introduces readers to the theory of dynamical systems through theoretical and practical examples, all illustrated with programs created with software tools from Maplesoft. These programs model phenomena such as chemical kinetics, electrical circuits, mechanical systems, and fractals.
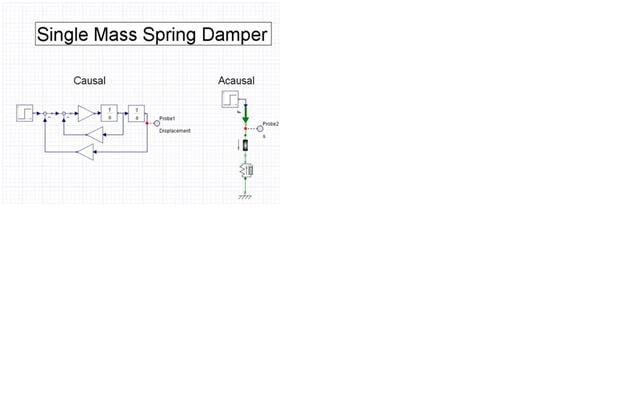
In a chapter devoted to continuous and discrete simulation, Dr. Lynch presents MapleSim as a tool for exploring chaos and the dynamical behavior of real systems without the complexity of more traditional simulation and modeling packages. The chapter introduces simulation concepts with the signal-flow solution of a resistor-inductor circuit modeled in Simulink. This is then contrasted with an acausal model of the same system using MapleSim. Dr. Lynch comments, “MapleSim is fantastic. Its representation looks a lot better. Students and scientists alike are more at home with the acausal representation of a circuit, and find it easier to pick up”. He says that MapleSim enables students to model real-life chaotic and dynamical systems at the physical component level and delivers an environment in which the parameter space can be explored rapidly and efficiently. This gives students greater appreciation of the mathematics that defines the dynamical behavior of everyday systems.
Dr. Lynch also introduces readers to the MapleSim Connectivity Toolbox. This newly released add-on product enables engineers and students to model systems at the physical component level in MapleSim, and then automatically generate a highly optimized S-function for instant use in Simulink.
The chapter then continues with models that explore the limit cycle of an underdamped oscillator, a periodically forced pendulum, and ends with a discussion of chaos control and synchronization.
Dr. Lynch has chosen to feature MapleSim in the second edition of his best-selling book, Dynamical Systems and Applications using Maple. Due to be published in the summer of 2009, the book introduces readers to the theory of dynamical systems through theoretical and practical examples, all illustrated with programs created with software tools from Maplesoft. These programs model phenomena such as chemical kinetics, electrical circuits, mechanical systems, and fractals.
In a chapter devoted to continuous and discrete simulation, Dr. Lynch presents MapleSim as a tool for exploring chaos and the dynamical behavior of real systems without the complexity of more traditional simulation and modeling packages. The chapter introduces simulation concepts with the signal-flow solution of a resistor-inductor circuit modeled in Simulink. This is then contrasted with an acausal model of the same system using MapleSim. Dr. Lynch comments, “MapleSim is fantastic. Its representation looks a lot better. Students and scientists alike are more at home with the acausal representation of a circuit, and find it easier to pick up”. He says that MapleSim enables students to model real-life chaotic and dynamical systems at the physical component level and delivers an environment in which the parameter space can be explored rapidly and efficiently. This gives students greater appreciation of the mathematics that defines the dynamical behavior of everyday systems.
Dr. Lynch also introduces readers to the MapleSim Connectivity Toolbox. This newly released add-on product enables engineers and students to model systems at the physical component level in MapleSim, and then automatically generate a highly optimized S-function for instant use in Simulink.
The chapter then continues with models that explore the limit cycle of an underdamped oscillator, a periodically forced pendulum, and ends with a discussion of chaos control and synchronization.

